Last week we looked at some different ways to plot the same data, with each chart enabling the reader to glean different information.
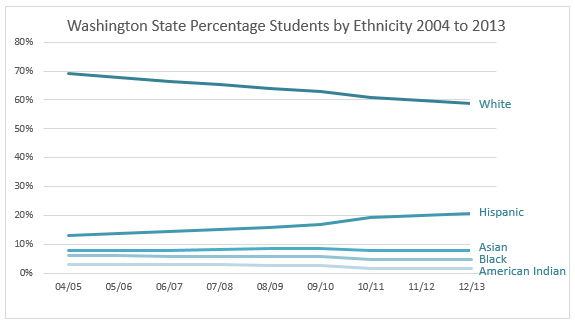
A by-product of that post was the observation that one series, the White, dwarfed the others, which made it difficult to get a feel for the trend and significance of the change for the smaller series, as you can see below.
Now, this isn’t so much of a problem if the purpose of the chart is to allow you to compare the size of each series to one another and their general trend over time. The line chart above does an ok job of this.
However if you want to get a feel for the magnitude of change over time for each series then the line chart makes you work harder than you should have to.
For example, the Hispanic population have increased by 55% in 2012/13 compared to 2004/05 but the line chart doesn’t easily convey this message.
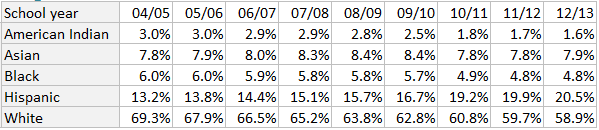
Let me be clear: in 2012/13 Hispanic Students account for 20.5% of the student population which is 55% more than 2004/05, which was 13.2%. Blimey those percentages of percentages can get confusing!
So, what charts can we use to emphasise this change?
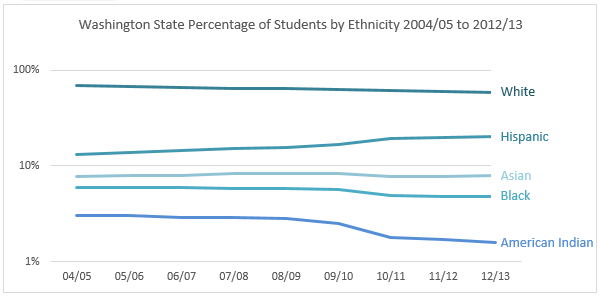
Option 1 – Logarithmic Scale
The logarithmic scale was born to present data that covers a large range of values like we have above (ok, ‘born’ might be a slight embellishment! I don’t think my high school math teacher would have described them like that).
The logarithmic scale uses intervals that correspond to orders of magnitude (in the chart above I’ve used a base of 10 to make it easier to understand, but 2 gives an even more defined change).
Each unit increase on the vertical axis represents an exponential increase in the underlying quantity. This effectively reduces the wide range of values to a more manageable size.
The Problem with Log Scales
They’re not intuitive to read and if you don’t have a math degree, or you were too busy passing notes in high school when they covered logs then you might make the wrong assumptions.
The bottom line is you need to know your audience when presenting charts that use log scales, or else be prepared to explain them.
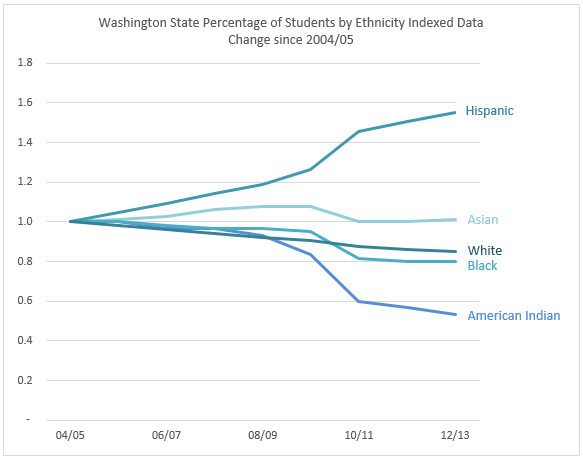
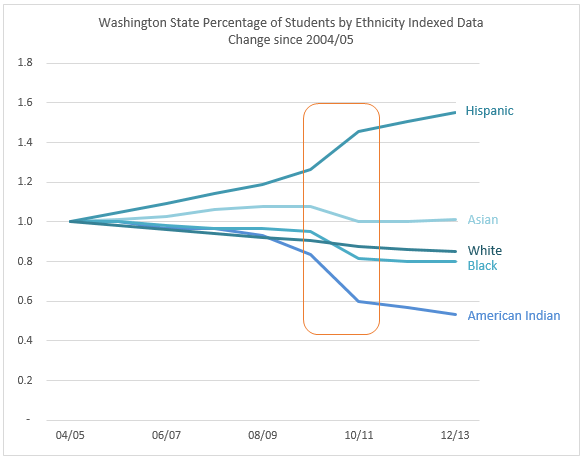
Option 2 – Indexing Change since 2004/05
This suggestion was sent in by Bryan Metz. Thanks, Bryan 🙂
Indexing enables us to see how much a group changes over time in relation to its starting point.
What we are essentially saying is that in 2004/05 every group starts at 1 or 100%. Any change below 1 indicates a reduction and any change above 1 is an increase since 2004/05. The number after the decimal place in the vertical axis is the percentage change.
Easy to read, eh?
Calculating the Index
We calculate the index by dividing each group’s percentages by its initial percentage in 2004/05.
For example, if we take the source data below for Hispanic Students we would calculate the indexed value for 2006/07 by dividing 14.4% by 13.2% which gives us 1.09, or a 9% increase in 2006/07 over 2004/05.
The Story it Tells
Interestingly in this chart we can see that in 2010/11 there is a spike in the degree of change and then it slows down again after that. This is not as clear in any of the other charts so far.
We can also quickly see that Hispanic Students have increased by nearly 60% since 2004/05, Asian increased for a while but are back at 2004/05 levels, White are on a steady decline, Black have reduced by 20% and American Indian by nearly 50%.
I like this method of indexing as it shows the trend over time. It’s intuitive; an upward line tells me the trend is upwards, likewise for the downward lines.
Another way to index is to do so year on year, or from one period to another, which brings us to our next option.
Smooth segue, eh? 🙂 Oh dear…. I think I spent too much time in Canada in December because ‘eh’ is becoming a habit.
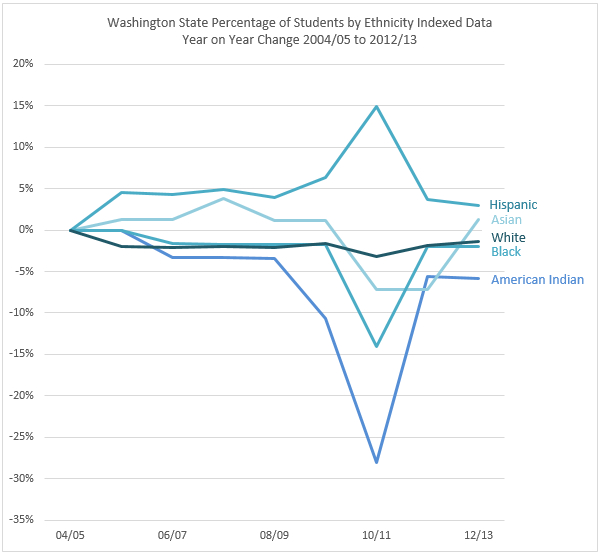
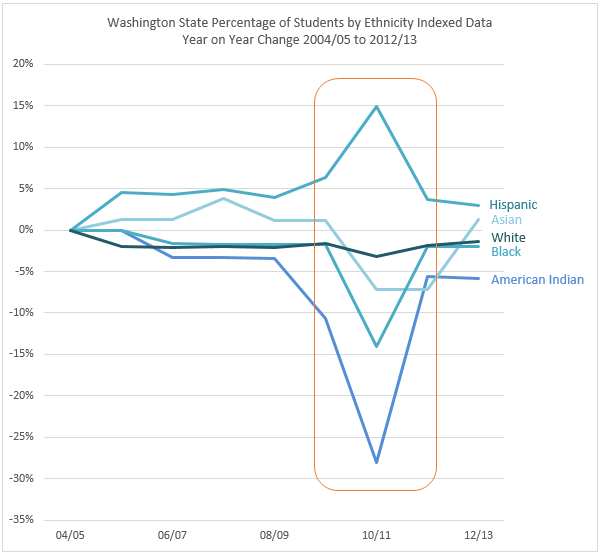
Option 3 – Indexing Change Year on Year
This suggestion was made by Duncan Williamson. Thanks, Duncan 🙂
Much like the previous chart, the total percentages have been indexed, however in this case they all start at 0%. A positive point on the chart indicates an increase on the previous year and a negative point indicates a decrease. So far, so good.
Calculating the Change
The indexing for each series in this chart is calculated by dividing the value for each year by the previous year to get the year on year percentage change.
For example the year on year change from 2004/05 to 2005/06 for Hispanic Students is =(13.8%/13.2%)-1 = 0.045 or a 4.5% increase.
The Story it Tells
This chart does a good job of highlighting the sudden change in 2010/11, and it does so more dramatically than the first Indexed Chart. It also clearly tells me the amount of growth each year, but for me it is less intuitive to read.
And it's because in this chart a relatively horizontal line above zero indicates a constant upward trend, and horizontal line below zero indicates a constant downward trend but I have to think about this to ‘see’ it (because it's horizontal), whereas the first indexed chart instantly ‘shows’ me with the direction of the line.
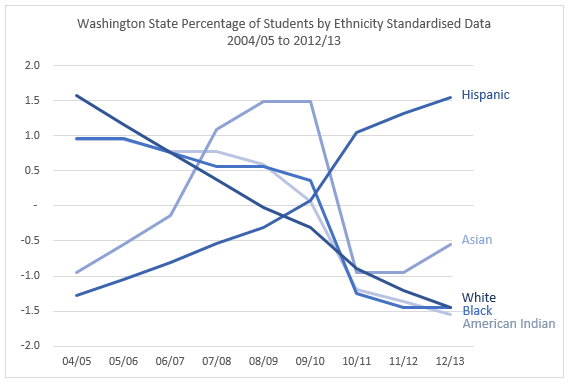
Other Options
There are some other methods for indexing, like standardizing or z-scores. There’s even a function in Excel to STANDARDIZE data, but when I experimented with these numbers the resulting mash of crazy lines looked like someone had thrown down a pile of Pick-up Sticks on my chart. You can see it here:
The angles of the lines are extreme, and for my liking they over emphasise the change. This risk with this chart is that the untrained chart reader will either panic (those lines look like serious problems), or switch off, as its meaning is too difficult to understand.
If you’re interested; the STANDARDIZE function takes the range of percentages for each series (ethnic group) and reduces them to a smaller scale by calculating a normalised value from a distribution characterised by the mean and standard deviation…..ZZZZzzzzz
Did you fall asleep? Sorry.
While z-scores have their place in statistics, I think they are best left for those with a Math Black Belt. For most of the data we’re likely to analyse the other options above will be more easily interpreted and relevant to our audience.
The Bottom Line
Just as in last week’s post, there’s no right or wrong chart. Each one has its own message. Which chart is best all depends on the question you are trying to answer and the audience you’re presenting to.
What about the Real Numbers
Bryan also said in his email to me:
“Comparing percentages is nice, and now that we've indexed it they are easy to compare, but they only tell part of the story. There's no way to tell why one race is increasing or decreasing relative to another. Are White student populations actually decreasing, or just not increasing as quickly as Hispanic populations (assuming, of course, that the total population is in fact increasing)? My second graph is a simple line graph of the absolute numbers (retrieved from the same source website). We hit the same issue here as with the initial graphs -- it's clear that the overall White enrollment is decreasing while the Hispanic is increasing, but the other populations are rather squished together.”
If you’d like to see Bryan’s additional analysis you can download the file here which also contains the charts above.
Enter your email address below to download the sample workbook.
Want More?
If you’d like to learn more about data visualisation and charts please consider my popular Excel Dashboard course.
I’ll teach you the fundamentals for data visualisation and I’ll show you the tricks I used to create the charts above, plus a load more.
And to give you a headstart download my free 30 Tips for Better Charts eBook here.
Thanks
Thanks again to Bryan and Duncan for sharing their charts and ideas.